1 Introduction

Any man-made or natural object is composed of smaller interacting units that define a whole. In a visual analysis of form, all the different units may be characterized by their size and other properties such as shape, texture, color, etc. For many years, it was assumed that these properties are either decided randomly, or depend entirely on the preference of the individual artist or architect. We argue that this is not the case at all, and identify a set of constraints that may be essential to design.

There exist rules, hitherto hidden in the design and assembly of complex structures, which impose constraints on how a form is put together. All of these rules can be found -- once one knows how to look for them -- in traditional art and architecture. The rules guarantee a connection to the human observer, who notices (albeit subconsciously) the mathematical ordering inherent in a pleasing design. For example, design units are very rarely single; they usually recur with some multiplicity. Another way of saying this is that the visual need for ordering leads us to make certain subunits similar to each other. The amazing thing is that nature also creates structural subunits that are similar.

This paper investigates one kind of hierarchical ordering, which is the distribution of subunits according to their size. This process is entirely distinct from the more obvious geometrical ordering of forms through symmetry -- as, for example, arranging elements along a line or in a reflected pattern -- yet it is just as important for the eventual coherence of the object, as perceived by a human being.

Architectural scales are defined by similar elements that repeat in a structure. Independent scales arise from the materials, structure, and functions, which distinguish particular scales that are essential to the structure's character. For example, a building might have several obvious levels of scale x_j . Each level j is fixed by the sizes x_j of similar, repeating elements. The structural scales begin with the form's overall size, and go down to about 3mm, if we do not take into account the microscopic levels of scale in the materials. To solve the problem of linearly measuring two and three-dimensional components, we use only a characteristic scale such as the width of a structural subunit.

Scales play a major, even if subconscious, role in design because they facilitate the process of human cognition. The mind of the observer groups similar objects of the same size into a single level of scale. This process, which has been compared with digital image compression in computers, reduces the amount of information presented to the observer by a complex structure. The mind apparently also estimates the number of similar objects on each scale, i.e., their relative multiplicity, and compares these numbers to what it knows regarding complexity from naturally occurring structures. If the distribution of scales and the relative multiplicity of elements correspond to an experientially generated internal standard, we perceive the structure as coherent.

An object's impact depends on the distribution of its subunits, independently of other features such as shape, form, and proportion. One comes back to the old question of what makes a complex structure interesting to human beings. The answer, at least in part, is that it has to lie somewhere in between two extremes: too regular or empty, which is boring; or too incoherent, which is disturbing1. Artifacts and buildings are as important for the pleasure they give as for any purely utilitarian function they serve. Just as in music, we enjoy a building or city because it offers a mixture of regularity and surprise in a certain ratio2. Regularity and surprise are complementary qualities that depend on how subdivisions are distributed.

2 The multiplicity rule for structural and design subdivisions

Drawing an analogy with allometric growth in biology, we propose a rule that governs the multiplicity of design elements on each level of scale. This result provides a means of balancing different design elements according to their size. When architectural elements are quantized into a number of distinct scales, they define a scaling hierarchy. The following suggestion for an optimal distribution of sizes enables one to analyze (and correct) flaws in existing designs, and leads to practical guidelines for creating original new designs.

Rule: The relative multiplicity p of a given design element, i.e., the relative number of times it repeats, is determined by a characteristic scale size x as roughly px^m = C , where C is related to the overall size of the structure, and the index m is specific to the structure. In general, m is restricted to the values 1 < m < 2 .

We will develop the motivation for and present evidence supporting this multiplicity rule. Remarkably, it turns out that the same rule is satisfied by many natural and social systems. West and Shlesinger3 argue that this is a universal rule for both natural and man-made structures.

A structure is usually said to be self-similar if it obeys the generalized hyperbolic relation,

px^m = constant

(1)

and, in addition, there exists a structural (geometrical) similarity between x at different magnifications. In artificial fractals, such as those studied in Section 6, below, a geometric pattern repeats exactly, whereas in natural objects it is the degree of complexity that repeats at different scales (sometimes referred to as statistical self-similarity). Here, we associate the relative multiplicity p in Equation (1) with a probability density, and will concentrate on the process that gives the distribution (1). We suggest the adoption of (1) as a universal scaling law in the design of complex systems, and justify its use subsequently.

The reader can now proceed to the applications in the rest of this paper (Section 7). Nevertheless, we are addressing the very basis of structural form, and it is useful to review just what sort of result we can expect. It is widely accepted that even the simplest complex system eludes the deterministic description of the physical sciences4. We cannot expect to describe a complex system by a field equation, because the mechanisms in complex systems are correlational rather than causal. The idea of causality is here replaced by the notion of concurrence, and the predictive relationships of physics are replaced by scaling relationships. Therefore, a scaling rule for complex systems is just as basic an organizing principle as an analytic law in physics. A field equation is usually stated in terms of analytic functions that obey an equation of motion. In a complex system, on the other hand, the best description is in terms of probability distributions.

Because of its practical importance, the following section presents an entirely distinct derivation of the multiplicity rule (1). We will show how it arises out of entropy considerations and the assumption that the generative process is independent of any particular scale.

3 Structural entropy, information, and the scaling hierarchy

An analogy with physical systems offers a way to measure the impact, or relative weight of each structural scale. To quantify this separation into scales we use the physical concept of entropy S [* see the complete version of this paper]. The question is how to choose the appropriate values of the set of multiplicities (probabilities), p_j . If we assume that we can measure certain quantities then the p_j must be consistent with those measurements.

This procedure is known as entropy maximization and it yields the most probable distribution consistent with the measurements. Stated differently, the system is organized around the measured averages and made maximally random otherwise. It measures equally the information of a system5. The ensuing derivation holds just as well for the information contained in a complex structure, as encoded in its substructure. We will later examine the importance of the informational interpretation.

We are going to derive several different distributions for the sizes of subelements in any complex structure. The exponential or Poisson distribution is consistent with a measurement of the first moment of the process. It depends on the existence of a preferred scale <x>. In the same way, one can find that the Normal or Gaussian distribution is consistent with a measurement of the second moment of the process, <x²>6. The emergence of order in complex systems, however, is fundamentally based on correlations between different levels of scale. The organization of phenomena that belong at each level in the hierarchy rules out a preferred scale or dimension. For this reason, neither the exponential (Poisson) nor the Normal distribution can describe morphogenesis that evolves through levels of scale.

What we face now is a determination of the distribution that is consistent with a scale-free process. In the context of perception, the human nervous system responds according to the logarithm of a signal rather than the raw signal itself (the Weber-Fechner law; 7). Note that this constraint is different from those usually imposed in that it is not just a moment, but a general property of the process.

After some algebra we determine that the normalized probability density is precisely of the form of the inverse power law (1). This is known as a hyperbolic or Pareto distribution, and has the form of a renormalization group relation. Our result implies that:

The smallest scales in the structure are intimately related to the largest scales in the structure and one cannot be changed without changing the other.

Another way to view this relation is that changes on any scale ripple through the entropy, affecting the overall impact of the structure on the viewer. The constraints were chosen in order to obtain a precise distribution function by which the different organizational levels j are linked.

We have thus found a means of transferring entropy so that it is distributed proportionately among all the available scales of a complex structure. In Shannon's information measure, this is precisely the state of maximum information8. A hyperbolic distribution (1) requires that smaller elements be more numerous than larger elements, but changes in scale as determined by the inverse power law are slow to decrease the contribution of the various scales to the structure. In other words, because the hyperbolic distribution's tail is broad, even the largest and smallest scales have a finite probability of contributing to the overall structure. By contrast, consider the Normal or Gaussian distribution in which the scales cluster around some mean value: as the probability of scales very different from this average value is essentially zero, they do not contribute to the structure.

Treating each level of scale as an independent entity, we have defined what corresponds to the structural entropy, or information, of the whole. The impact a design has on a viewer depends on the size of its features, and on their multiplicity (how many elements are of the same size and therefore how much information is associated with that scale). As with physical quantities, the structural entropy is additive, so the total impact of a particular design is due to the sum of all the separate contributions to the entropy from each of its scales.

The more levels of scale a building or city possesses, the more structural entropy (information) it will have, so that subdividing a form generates structural entropy (information). This result is known from information theory, as one way of increasing the information of a system consisting of equally likely choices is to increase their number 9. Some optimum value of S will depend on geometrical constraints, and there is a cutoff at the smallest perceivable size. In an organized system with balanced internal components every entropy mode should have proportional weight so that the overall structure is scale-free. Departures from entropy balance are felt just as strongly as departures from geometric balance (as in a deconstructivist building). The total structural entropy S is proportionately distributed when any two scales i and j have equal contributions S_i = S_j to the total entropy S. From information theory, this is precisely the condition giving maximum information for the whole10.

We have derived a multiplicity rule for sizes. This should not be treated as a rigid rule: it indicates the approximate relative number of elements that ought to be defined on different scales. For perceptual balance, a design should have relatively many sets of small similar design elements, and relatively fewer large elements in a proportion that does not favor any one scale over any other. Equation (1) holds regardless of the size of the structure. In buildings and cities, it is satisfied by a majority of architectural and urban styles (though not those of the twentieth century). 

4 The necessity for distinct scales, or the quantization of design subdivisions

Our rule for the multiplicity of design subdivisions presupposes the existence of distinct levels of scale. The multiplicity rule depends upon repeating elements of the same size. Clearly, a random distribution in the sizes and shapes of subelements will frustrate their grouping into distinct scales, leaving many scales with very low multiplicity. Therefore, an important part of a structure's design coherence rests on the quantization of its scales. This leads us to another concern -- the actual spacing between levels of scale -- which is discussed elsewhere11.

Scaling coherence is reduced when: (a) forms are empty and have few or no subdivisions, and (b) random forms have subdivisions that do not repeat. The first instance provides no elements with which to define any sort of scaling. It is impossible to relate the large scale to the small scale without the existence of intermediate scales; the conceptual gap is simply too large. The second instance imposes the opposite problem: human cognition is frustrated because the mind cannot group the different elements together into scales. This case leads to information overload, which is just as disturbing as the first case.

What we need to show is that the form of scaling provided by the multiplicity rule satisfies the needs of the observer by properly scaling the intervals between the available sizes. This means that since not all scales are present within a given scheme (which would represent an enormous amount of information), the information absorbed by the observer is manageable because there is only a discrete number of scales, and each scale that is present conveys the same amount of information as every other scale.

We can plot the distribution of levels of scale in an actual building or artifact using a logarithmic scale for the dimensions of design elements x on the horizontal axis, and the logarithm of their multiplicity pon the vertical axis. Performing these measurements will provide a distribution graph of lnp versus lnx , showing how the elements of different scales contribute in the total design or structure.

To compare such a graph of lnp versus lnx to the theoretical prediction, take the logarithm of the multiplicity rule, (1) to obtain:

lnp = -mlnx + lnC

(2)

so that on log-log graph paper the slope of the curve relating lnp to lnx is the exponent of x , and the constant is the intercept on the vertical axis. Sample measurements reveal a consistent distribution of subelements satisfied by objects that give emotional pleasure. Points plotted on such a graph lie approximately on a straight line with a slope close to the theoretical prediction. In many cases the slope is given by negative one, m = 1, which would correspond to an inverse-power 1/f distribution12.

One also sees a quantization of scales13. This is equally important, though it is not necessarily a consequence of the inverse power law form of the distribution. In general, data points are not evenly distributed on the log-log plot, but are clustered into discrete groups separated by gaps. In the majority of cases, the distribution very clearly follows the theoretical rule of one cluster of points for each integer on the horizontal axis, which is to say, equally spaced collections of measurements on a logarithmic scale. Said differently, if we express the multiplicity as a function of the size using the multiplicity rule, then scaling the size by a factor k yields

p(kx) = k^-mp(x)

(3)

In the case where m = 1 Salingaros14 argues that the scales are separated by a natural scale value k = e = 2.718.

5 The same rule is ubiquitous

Consider the numerical distribution of animals in a natural habitat. Intuitively, we expect to find more smaller than larger animals, and that is indeed the case. One can measure the population density of a particular type of animal, and correlate it with its mass. The relationship between body mass (a general characteristic of size) x , and the relative abundance p, of different species of animals in an ecosystem is precisely that given by (1); see, for example, Bonner15 and Peters16. All the data, from invertebrates to mammals, can be described by a straight line on a log-log plot with slope equal to -1 corresponding to m = 1.

In general, we have m not equal to 1 for other systems, although the departures are small. One is faced with an enormous number of instances in which the multiplicity rule applies; we mention some of them below to indicate its universality. The application of (1) to natural and social phenomena appears to be endless and can apparently be traced to the self-similar behavior of complex structures and processes17.

The multiplicity rule is observed if p is the probability of having an income of size x then 1.5 < m < 2, see Pareto18; if p is the relative number of cities having a population of a given size x then m = 1 , known as Auerbach's Law, see Lotka19; if p is the relative number of genera in a species and x is the rank order of those genera then 1 < m < 2, see Willis20 or Lotka21; if p is the relative frequency of the usage of a word in a language and x is the rank order of that word then 1 < m < 1.5, see Zipf22; if p is the relative frequency in the number of authors of a given number of papers published in a year x then m = 2, see Lotka23; if p is the relative number of purines in a DNA sequence and x is the difference in the number of purines and pyramidines then m = 1.25, see Allegrini et al24. There are many more examples from the sciences, geography, music, and economics that we could cite.

Each of these examples is generated by different physical processes, and in some instances, the generative mechanism is not well-understood. Nevertheless, all those distinct processes lead to the same mathematical rule: this shows a remarkable convergence. In those poorly-understood cases, the observed morphology may offer the only basis for trying to analyze a highly-complex mechanism.

6 Fractals and the multiplicity rule

The multiplicity rule is linked in an essential manner to fractal geometry. Fractals are characterized by a difference between their topological and fractal dimensions. That is, a fractal line has topological dimension 1, but it could have infinite length and so be space-filling. Its fractal dimension in fact corresponds to our index m , which for a fractal line takes values 1 < m < 2. This will be shown explicitly for two well-known examples; for the figures, see Batty and Longley25, Mandelbrot26, or West and Deering27.

6.1 The von Koch snowflake 

This classic fractal possesses a natural ranking of decreasing lengths into distinct scales. Starting from an equilateral triangle with unit side, we generate new corners in the middle of each side using a scaling factor r = 1/3. Subdivide each side into three equal parts, and use the middle section as the basis of an equilateral triangle of side 1/3, pointing outwards. Repeat this process indefinitely. Call the (unnormalized) number p_j of lengths x_j . With the initial values p₀ = 3, x₀ = 1, it is easy to show that:

p_j = 3.4^j , x_j = 1/3^j

(4)

To avoid confusion, note that here, increasing j corresponds to decreasing x , which is the opposite of other models with scaling. Using (4), the quantity p_jx_j^m is finite and equals 3 for any j , with m equal to the fractal dimension,

m = D_H = ln4/ln3 = 1.26

(5)

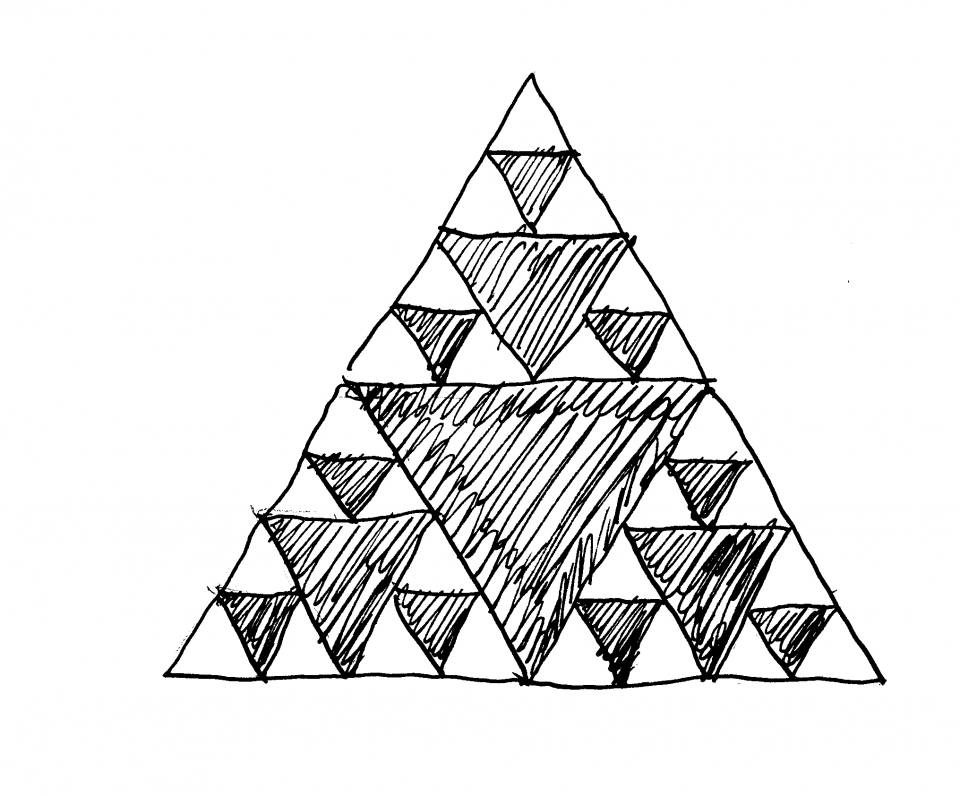
6.2 The Sierpinski gasket 

Again, we begin with an equilateral triangle of unit side, and insert progressively smaller triangles into its interior, this time using the scaling factor r = 1/2 . With p₀ = 3 , x₀ = 1 , we find for the jth iteration:

p_j = 3^j+1 , x_j = 1/2^j

(6)

The constant measure is again p_jx_j^m = 3 , when the index m is equal to the fractal dimension,

m = D_H = ln3/ln2 = 1.58

(7)

These two examples serve to link the derived multiplicity rule to the theory of fractals. The multiplicity rule is a more general condition than fractal scaling. There is a debate about what to include in the definition of a fractal. Many instances of the multiplicity rule have m = 1 , whereas fractals are usually understood as having fractal dimension m > 1 (for a fractal line). The value of the fractal examples is that they show discrete hierarchies, because they are self-similar with some scaling factor r . The plot of lnp versus lnx is discrete with points evenly spaced along a straight line. A hierarchical quantization of sizes is an important consequence of the self-similarity of these two examples.

This paper could simply have offered a restatement of the power-law self-similar scaling relation in ideal fractal geometry. Our multiplicity rule (1) then follows without further assumptions. Nevertheless, there are crucial differences. First, natural fractals are not the artificial self-similar fractals that we analyzed above. Statistical self-similarity, as is found in natural structures, does not give an exact scaling relation. Second, we have derived the scaling relation from an approach that does not use fractal concepts. This derivation is therefore more general, and more believable, than merely asserting that urban structure should follow fractal scaling. Why should it? The fact that it does is a consequence of fundamental structural laws that are also responsible for the fractal qualities of many naturally-occurring structures.

One must not be misled by the high symmetry of the above fractals, as the multiplicity rule is independent of any symmetry. This is the point made in Salingaros28, where scaling and symmetry are distinguished. Stochastic fractals such as a Lévy flight consisting of connected independent lengths distributed according to a homogeneous power law obey a multiplicity rule such as (1)29. Those cases have no symmetry. Some of the examples given in the preceding section correspond to stochastic fractals.

7 How violating the multiplicity rule destroys a city

We are going to discuss three cases relevant to urbanism: (i) the distribution of path lengths or widths; (ii) the distribution of budgets for urban construction and repair; and (iii) the distribution of urban elements according to size. In all three instances, modern cities violate the inverse power-law scaling. By now there is sufficient evidence to suggest that these violations may be in large part responsible for the perceived inhumanity of urban regions, which in turn contributes to the decay of our cities.

Among the key questions in urban morphology is: "What is the most important scale in a city?" The answer is negative:

There is no predominant scale in a city, because a city is a complex hierarchical system.

Human activity processes have to occur over an enormous range of scales, and each of these determines the scale of built structures. The distribution of substructures therefore has to be scale-free, which implies a hyperbolic or inverse power-law distribution. 

7.1 Distribution of path lengths and widths 

Recent investigations into the connectivity of urban form30 lead us to conclude that a functioning urban fabric -- a living neighborhood -- is connected by paths that obey an inverse power-law distribution. The most successful urban regions all over the world are found to have a great range of connections, from footpaths, to bicycle paths, to low-traffic roads, to through roads, up to expressways; in decreasing number. Urban connections may sometimes be characterized by their width rather than their length. The data comes indirectly from measuring the fractal structure of urban connective networks, which implies an inverse power-law distribution according to both the length and width of individual paths (corresponding to the intensity of traffic flow).

This finding contrasts with dysfunctional urban regions, which represent connective networks with a peaked distribution. At one extreme we have the modernist city and suburb, which lack small-scale connections31. Planners focus on highways and middle-density roads. The arbitrary design of urban elements that emphasizes the large scale makes low-density roads and paths on the human scale difficult or impossible to include. By eliminating the pedestrian path network of older cities, one loses the interactivity present in historical neighborhoods. At the other extreme, the inner-city ghetto or squatter settlement lacks longer connections because of socioeconomic conditions and the availability of jobs, and not from the road structure, and this isolates its residents from the rest of the city and from society in general. Again, a skewed distribution in the length of effective paths disconnects the inner city from the whole.

Independent support for this result comes from the study of neural networks. Networks that appear in both natural and artificial systems -- such as the nervous system of some invertebrate animals -- contain ordered near-neighbor links as well as random links of much longer length. It was recently shown how the two extremes (a regular network of only short links on the one hand, or a totally random network of mostly longer links on the other) both have drastically reduced global connectivity properties compared to the case where the path lengths are distributed more according to an inverse-power multiplicity rule32.

A mathematically modest transformation can alter the global connectivity properties of a network. A regular network -- which has only nearest-neighbor interactions -- can be changed by disconnecting a small number of near links, and replacing them with random longer links. This procedure leads to a "small-world" network33. This new object has features that are shared by neither totally regular, nor by totally random, networks. Random graphs -- which are completely connected -- will have a distribution of different path lengths, with a peak not far from that of an exponential distribution. Especially relevant to urbanism is the converse process of replacing a few longer, random connections, by many nearest-neighbor connections. We are conjecturing that "small-world" networks in fact follow an approximate inverse-power distribution of connection lengths. Rather than being the result of a randomization process, they are a redistribution into a definite, more stable global state.

7.2 Distribution of project funding in urban construction

A remarkable discovery of Alexander and his associates relates to what happens when money for building projects is distributed according to different lawsAlexander et. al., 1975, op.cit.; page 95. The optimum distribution for the allocation of funds between different projects for any given time period is to give equal budgets for several (say, five) different categories according to increasing size, distributed as in (1). If x is the size of the project (which is roughly proportional to its cost) and p the number of projects of that size, then m = 1. Apparently unaware of inverse power-law scaling, Alexander and his colleagues derived the rule from looking at many instances -- successful as well as unsuccessful -- of urban growth.

A large lump development includes large projects, but very few medium and small projects. The total amount of money allocated invariably nowadays goes to these large projects, and the larger the project, the more chance it has of being funded. This situation destroys the urban fabric, for the following reason. Ongoing repair of the fabric also requires the allocation of funds for a large variety of projects on all the intermediate levels of scale, and most importantly, for an enormous number of very small projects. What happens in practice is that the giant projects eat up all the available money, and therefore leave nothing to be spent on smaller and intermediate size construction. Without repair, the entire city decays.

A funding distribution skewed heavily towards the large scale gives rise to a particular philosophy of urban growth. By ignoring the small and intermediate scales, urban actions become interventions, and then turn exclusively to the large scale. Any urban solution is erroneously believed to succeed only on the largest scale. Repair of existing buildings is deemed unimaginative or uneconomic, and piecemeal growth by adding successively to existing structures is not even seriously considered. The organic growth of cities, such as occurred for millennia to generate the best-loved urban regions all over the world, is ruled out. This philosophy has transformed our cities by replacing their natural, fractal structure with enormous, unlivable apartment blocks and unused urban plazas.

7.3 Distribution of urban elements according to size

Traditional cities and towns contain urban elements of many different sizes; from the largest buildings down to street furniture, bollards, and potted plants. We claim that a necessary though not sufficient condition for a living city is that urban units be distributed according to the inverse power-law scaling (1). The larger buildings and open spaces should be few, and increase in number as their size decreases. Most important, there must be smaller urban elements, in increasing numbers, down to the human scale. These include clearly-defined subdivisions of larger units, as well as separate autonomous structures. The hierarchy does not stop there, however, but should continue through architectural scales in buildings, into the structural scales found in natural materials34.

Today's cities follow stylistic rules that skew the distribution of urban units towards the largest possible scale, which is irrelevant to human activity. The intermediate scales are severely weakened. Worst of all, the explicit design goal of "cleaning up" the geometry of cities has totally eliminated the smaller urban elements. The modernist vision of megatowers set in enormous parks represents a fundamental violation of natural scaling laws. This affects much more than visual appearance. A skewed distribution in the sizes of urban elements makes it impossible to generate the appropriate connections that tie a living city together (thus causing the problem with path lengths and widths discussed above). Third-world countries wishing to modernize apply the deceptively simple modernist model, and unintentionally destroy their cities.

Another culprit is suburban sprawl. Single-family houses of roughly the same size, with their corresponding front lawns, create a peak in the distribution of urban elements at the size of a single house unit. Overall, we have two peaks in the size distribution of components in a contemporary city, one corresponding to giant office and apartment buildings, and the other corresponding to suburban houses. There is relatively little of intermediate size, and almost nothing smaller than a suburban house that forms a coherent piece of the city. This contrasts sharply with the living urban fabric as measured in historic regions of cities in developed nations, as well as in indigenous cities of the third world35.

8 Verification in art and architecture

The results of this paper provide a measure by which artistic, architectural, and urban trends may be evaluated. Most design innovations are resisted at first, and many (though not all) are eventually accepted precisely because they provide the same subconscious pleasure from perceptive input as more traditional forms. If, as we suggest here, this has to do with hierarchical scaling and the distribution of information among available scales, then we can claim a commonality for many diverse yet appealing design styles. We further conjecture that the reason why certain design innovations are not widely accepted, even after a period of familiarity, may be that they violate the multiplicity rule.

Nature was heavily relied upon as a source for design ideas up until the twentieth century. While artists and architects did not know about scaling laws for design, they knew the overall structure of plants, animals, crystals, and the human body. The different scales that people could observe in nature (and especially how they relate to each other) gave them an instinctive feeling for the scaling hierarchy, which they then applied to art and architecture. This is not conscious imitation of forms, but it still imitates the partitioning and structural complexity of natural structures.

We have now a basic confrontation between two world views. Design theories of this century are based on criteria having to do either with the purity, or with the novelty of forms. A point of view taught nowadays in schools of art and architecture is that it is perfectly valid to abandon a natural scaling law, precisely because that step gives rise to new forms that look different from traditional man-made objects. To contemporary artists and architects, the negative consequences on the observer or user are not an issue; indeed, using shock value validates an artwork or building as being even more novel. Scientific concerns are irrelevant in this world view, which is a major cause for alarm.

Nevertheless, an established "new" artistic form such as cubism, which when it was introduced appeared to fall into the above category of violating a natural scaling law, in fact does not entirely violate it. If one measures the distribution in the breakup of space, which is to say the distribution in the scale sizes used in Picasso's line drawings, we find the multiplicity rule36. In a study of twelve Picasso drawings via the box-counting method, a fractal dimension near m = 1.6 was found for the intermediate and large scales, although the small scales are weak. Etchings by Dürer, Rembrandt, and Munch, on the other hand, have a higher fractal dimension m = 1.8 to 1.9 right down to the smallest detail37.

Similar measurements have been conducted for architecture and urbanism. Living cities are found to have an intrinsically fractal structure38, which links them to the derived multiplicity rule (see Section 6 above). The fractal structure of traditional vernacular buildings on the coast of Turkey was measured by Bovill39 using the box-counting method to find a fractal dimension around m = 1.6. Bovill has also measured fractal dimensions for two of Frank Lloyd Wright's houses (Robie house and Unity Temple) and a casement window from the Robie house, again obtaining figures close to m = 1.6 over several different scales. By contrast, a similar analysis of Le Corbusier's Villa Savoye reveals no fractal structure40.

One may not unreasonably claim that our response to form and structure has a physiological underpinning, and must therefore be appreciated on those terms. Such scaling laws in physiology are indicative of healthy organisms and the signature of pathology is the deviation of the power-law index from pre-established normal values. A dramatic confirmation of this is the observation of departures from the scaling rule with the onset of pathology. The power-law index is a measure of the degree of variability in the underlying process: too much variability and the organism cannot compensate, too little variability and the organism dies41. 

9 Conclusion

We derived a multiplicity rule for the distribution of sizes in architectural and urban elements that agrees with empirical observations. The levels of scale in a design are defined by elements of size x repeating a relative number of times p . The approximate rule px^m = constant follows from an analogy with the use of entropy as an organizing principle in physics. The index m typically takes on values between 1 and 2. This principle, coupled with the idea of designs that are free of dominant scales, leads to the inverse power-law distribution, which is found in many natural and man-made structures. Smaller elements are thus more numerous than larger elements, with a fixed balance of distribution between sizes.

Two very different derivations of the multiplicity rule were given: the first follows as a consequence of allometric growth; the second from a variational calculation that determines a scale-free process. We interpreted this result as the only means of transferring entropy or information so that it is distributed proportionately among all the available scales of a structure. This contrasts with other distributions in which a single scale dominates, and, as a consequence, contain less information. The derived multiplicity rule is obeyed by self-similar fractal structures, where our index m equals the fractal dimension D_H . We illustrated this correspondence with two well-known fractals, the von Koch snowflake, and the Sierpinski gasket.

The multiplicity rule was applied to discuss three separate aspects of urbanism: (i) the distribution of path lengths and widths; (ii) the distribution of project funding; and (iii) the distribution in the size of urban units. We argued that the multiplicity rule is found in, and actually generates and maintains traditional cities. By contrast, the modernist city grossly violates the multiplicity rule, and urban practices today perpetuate this violation. We suggested that this could be a major reason for the perceived lack of human qualities in contemporary cities, and could even contribute to their decay.

By applying the rules of scientific analysis we may have derived a link between certain ordering mechanisms inherent in the human mind and the structures we design. Some of our rules are apparently hardwired so we resonate with structures in which we recognize the same type of ordering. This idea is consistent with previous authors' arguments regarding the nature of beauty and aesthetics. In this view design and structure are not arbitrary, but have to satisfy a set of constraints. The organizational mechanisms underlying design were related here to analogous processes taking place in other complex systems in biology, economics, physics, and physiology. In this setting architecture and urbanism can profit from results already established in other disciplines. We thus provide a new framework in which to derive practical design rules based on what may well be invariant universal principles.

• 1. Salingaros, N. A., 1997 "Life and Complexity in Architecture From a Thermodynamic Analogy", Physics Essays 10 165-173.
• 2. West, B. J. and Shlesinger, M., 1990 "The Noise in Natural Phenomena", American Scientist 78 40-45.
• 3. ibid.
• 4. West, B. J. and Salk, J., 1987 "Complexity, Organization and Uncertainty", European J. Operations Research 30 117-128.
• 5. Shannon, C. E. and Weaver, W., 1949 The Mathematical Theory of Communication. (University of Illinois Press, Urbana)
• 6. West and Salk, 1987,op. cit.
• 7. West, B. J. and Deering, W., 1995 The Lure of Modern Science: Fractal Thinking (New Jersey: World Scientific)
• 8. Shannon and Weaver, 1949 op. cit.
• 9. ibid.
• 10. ibid.
• 11. Salingaros, N. A., 1995 "The Laws of Architecture from a Physicist's Perspective", Physics Essays 8 638-643; Salingaros, N. A., 1998a "A Scientific Basis for Creating Architectural Forms", Journal of Architectural and Planning Research 15 283-293.
• 12. West and Shlesinger, 1990, op.cit.
• 13. Salingaros, 1995; 1998a, op.cit.
• 14. ibid.
• 15. Bonner, J. T., 1988 The Evolution of Complexity by Means of Natural Selection, (Princeton, New Jersey: Princeton University Press)
• 16. Peters, R. H., 1983 The Ecological Implications of Body Size (Cambridge: Cambridge University Press)
• 17. West and Deering, 1995, op.cit.
• 18. Pareto, V., 1897 Cours d'Economie Politique (Lausanne, Switzerland)
• 19. Lotka, A. J., 1956 Elements of Mathematical Biology (New York: Dover)
• 20. Willis, J. C., 1922 Age and Area: A Study in Geographical Distribution and Origin of Species (Cambridge, England: Cambridge University Press)
• 21. Lotka, 1956, op.cit.
• 22. Zipf, G. K., 1949 Human Behavior and the Principle of Least Effort (Cambridge, Massachusetts: Addison-Wesley)
• 23. Lotka, A. J., 1926 "The Frequency Distribution of Scientific Productivity", J. Washington Academy of Sciences 16 317-323
• 24. Allegrini, P., Barbi, M., Grigollini, P. and West, B.J., 1995 Physical Review E 52 5281-5296
• 25. Batty, M. and Longley, P., 1994 Fractal Cities. (Academic Press, London)
• 26. Mandelbrot, B. B., 1983 The Fractal Geometry of Nature (New York: Freeman,)
• 27. West and Deering (1995), op.cit.
• 28. Salingaros, N. A., 1995 "The Laws of Architecture from a Physicist's Perspective", Physics Essays 8 638-643
• 29. West and Deering, 1995, op. cit.
• 30. Batty, M. and Longley, P., 1994 Fractal Cities. (Academic Press, London); Batty, M. and Xie, Y., 1996 "Preliminary Evidence for a Theory of the Fractal City". Environment and Planning A 28 1745-1762; Frankhauser, P., 1994 La Fractalité des Structures Urbaines. (Anthropos, Paris); Salingaros, N. A., 1998b "Theory of the Urban Web". Journal of Urban Design 3 53-71
• 31. Salingaros, 1998b, op.cit.
• 32. Watts, D. J. and Strogatz, S. H., 1998 "Collective Dynamics of 'Small-World' Networks". Nature 393 440-442
• 33. ibid.
• 34. Salingaros, 1998a, op. cit.
• 35. Batty and Longley, 1994; Batty and Xie, 1996; Frankhauser, 1994, op. cit.
• 36. Nyikos, L., Balazs, L. and Schiller, R., 1994 "Fractal Analysis of Artistic Images: From Cubism to Fractalism", Fractals 2 143-152.
• 37. ibid.
• 38. Batty and Longley, 1994; Batty and Xie, 1996; Frankhauser, 1994, op. cit.
• 39. Bovill, C., 1996 Fractal Geometry in Architecture and Design. (Birkhäuser, Boston)
• 40. ibid.
• 41. West and Deering, 1994, op. cit.